The Cyclotron

 

Erenst O Lawrence was a brilliant American nuclear physicist who is credited with the invention of the cyclotron, the compact particle accelerator, which is still used in nuclear physics laboratories. The cyclotron, despite being eclipsed by its larger, cumbersome cousins, the linear accelerators, can energize sub-atomic particles fairly easily while saving the space, normally taken by the latter. Ernest Lawrence won the Nobel Prize for physics in 1939 for the remarkable invention.

 

The cyclotron is a device that can accelerate sub-atomic particles to a very high speed for particle experiments and clinical needs. It energizes particles, sending them through an alternating electric field - while sending the particles in question in circular orbits with the aid of a magnetic field.

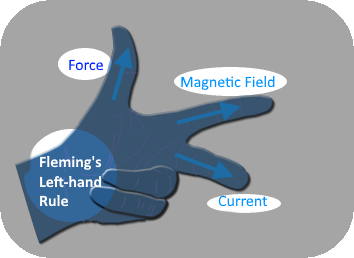
When an electron passes through an electric field at right angles, its speed increases - and so does its kinetic energy. If the electron in question, on the other hand, passes through a magnetic field, the former follows a curved path without its energy being changed, obeying Fleming's Left-hand Rule. Therefore, an electric field can be combined with a magnetic field - to energize an electron and change its path respectively.

This is what takes place inside cyclotron.

 

The D-shaped semi-circular arcs, known as Dees, are combined with a small gap between the two. A magnetic field acts at a right-angle to each dee.

When an electron is produced at the centre of the path between the two dees, it is attracted to the dee that is maintained as positive at that moment. The electron, once inside the dee, takes a curved path due to the magnetic field that acts through it - according to the motor effect. When the electron reaches the gap again, the polarity of the dees are reversed so that the electron is attracted to the second dee. The process continues up until the electron gains enough energy for subsequent nuclear experiment planned by the professional who uses it. The energized electron gets out of the outlet attached to the dees - at a very high speed. The energized electron can then be siphoned off for any particle experiments.

In linear accelerators, such as the LHC at CERN in Switzerland, circular paths are specifically constructed to energize the electron while sending them through electric fields for greater energies. The paths are often very long - up to 20 km.

Inside linear accelerators, the speed of the particles reaches up to 99.9% of the speed of light - without exceeding the speed of light. At such a high speed, relativistic effects set in: the increase in energy of the particles, leads to mass being increased, which in turn delays the arrival of the charged particles to where they originally emerged. As a result, a cyclotron can energize a charged particle only up to 1Gev. This limitation, however, has been addressed in synchrotron, which uses a fixed orbit while increasing the frequency of an alternating voltage.

In cyclotron, the speed of particles may not reach that speed. Its compact size, however, compensates for the loss of speed.

The particles accelerators are used to energize the sub-atomic particles so that they can be used in the experiments involving high-energy particles. They are used in medical experiments too.

 

 

If the charge of the particle inside a cyclotron, velocity of it and the magnetic flux density are q, v and B respectively,
The radial force on the charge = Bqv
If the radius of the circular path taken by the charged particle is r, the mass of the particle is m and the centripetal force - F_c - then,
F_c = mv²/r
The force on the charged particle is the centripetal force
So, Bqv = mv²/r
v = Bqr/m
If the time period of the particle is T,
T = 2πr/v
T = 2πm/Bq
If the frequency is f, since f=1/T
f = Bq/2πm

So, the frequency of the charged particle does not depend on the radius of the circular path; it just depend on the flux density, charge and mass of the particle.